What are reduction formulas? Reduction formulas
And one more point: there are quite a lot of reduction formulas in number, and we will immediately warn you against learning them all by heart. There is absolutely no need for this - there is one that allows you to easily apply reduction formulas.
So, let's write down all the reduction formulas in the form of a table.
These formulas can be rewritten using degrees and radians. To do this, just remember the relationship between degrees and radians, and replace π with 180 degrees everywhere.
Examples of using reduction formulas
The purpose of this paragraph is to show how reduction formulas are used in practice to solve examples.
To begin with, it is worth saying that there is an infinite number of ways to represent an angle under the sign of trigonometric functions in the form and 
. This is due to the fact that the angle can take any value. Let's show this with an example.
For example, let's take the angle under the sign of the trigonometric function equal to . This angle can be represented as 
, or how 
, or how 
, or in many other ways.
Now let's see what reduction formulas we will have to use depending on the representation of the angle. Let's take .
If we represent the angle as , then this representation corresponds to a reduction formula of the form , from which we obtain 
. Here we can indicate the value of the trigonometric function: .
For presentation we will already use a formula of the form 
, which leads us to the following result: .
Finally, since the corresponding reduction formula has the form 
.
To conclude this discussion, it is especially worth noting that there are certain conveniences when using angle representations in which the angle has a value from 0 to 90 degrees (from 0 to pi in half radians).
Let's look at another example of using reduction formulas.
Example.
Using reduction formulas, represent through the sine and also through the cosine of an acute angle.
Solution.
To apply the reduction formulas, we need to represent an angle of 197 degrees in the form or 
, and according to the conditions of the problem, the angle must be acute. This can be done in two ways: 
or . Thus, 
or 
.
Turning to the corresponding formulas for reducing and , we obtain and .
Answer:
And 
.
Mnemonic rule
As we mentioned above, it is not necessary to memorize reduction formulas. If you look at them carefully, you can identify patterns from which you can obtain a rule that allows you to obtain any of the reduction formulas. He is called mnemonic rule(mnemonics is the art of memorization).
The mnemonic rule contains three stages:
It’s worth saying right away that to apply the mnemonic rule you need to be very good at identifying the signs of sine, cosine, tangent and cotangent by quarters, since you will have to do this constantly.
Let's look at the application of the mnemonic rule using examples.
Example.
Using a mnemonic rule, write down the reduction formulas for 
And 
, considering the angle to be the angle of the first quarter.
Solution.
We don’t have to do the first step of the rule, since the angles under the signs of trigonometric functions are already written in the required form.
Let's determine the sign of the functions And . Provided that - the angle of the first quarter, the angle 
is also the angle of the first quarter, and the angle 
- angle of the second quarter. The cosine in the first quarter has a plus sign, and the tangent in the second quarter has a minus sign. At this stage, the required formulas will have the form and . Now that we have figured out the signs, we can move on to the final step of the mnemonic rule.
Since the argument of the cosine function has the form , then the name of the function must be changed to cofunction, that is, to sine. And the tangent argument has the form , therefore, the function name should be left the same.
As a result we have 
And . You can look at the table of reduction formulas to make sure that the results obtained are correct.
Answer:
And .
To consolidate the material, consider solving an example with specific angles.
Example.
Using a mnemonic rule, reduce to trigonometric functions of an acute angle.
Solution.
First, let's imagine the angle of 777 degrees in the form necessary to apply the mnemonic rule. This can be done in two ways: or.
The original angle is the first quarter angle, the sine for this angle has a plus sign.
For presentation, the name of the sine must be left the same, but to present the type, the sine must be changed to cosine.
As a result, we have and .
Answer:
And .
To conclude this point, consider an example illustrating the importance of correctly representing an angle under the sign of trigonometric functions for applying the mnemonic rule: the angle must be sharp!!!
Let's calculate the tangent of the angle. In principle, by referring to the material in the article values of sine, cosine, tangent and cotangent, we can immediately answer the question of the problem: 
.
If we represent the angle as or as , then we can use the mnemonic rule: 
And 
, which leads us to the same result.
But this is what can happen if you take a representation of an angle, for example, of the form. In this case, the mnemonic rule will lead us to this result. This result is incorrect, and this is explained by the fact that for the representation we did not have the right to apply the mnemonic rule, since the angle is not acute.
Proof of reduction formulas
Reduction formulas reflect periodicity, symmetry, and shift properties by angles and . Let us immediately note that all reduction formulas can be proven by discarding the term in the arguments, since it means changing the angle by an integer number of full revolutions, and this does not change the values of trigonometric functions. This term serves as a reflection of periodicity.
The first block of 16 reduction formulas follows directly from the properties of sine, cosine, tangent and cotangent. It's not even worth dwelling on them.
Let's move on to the next block of formulas. Let's prove the first two of them first. The rest follow from them. So, let’s prove the reduction formulas of the form 
And 
.
Let's consider the unit circle. Let the initial point A, after rotation by an angle, go to point A 1 (x, y), and after rotation by an angle, to point A 2. Let's draw A 1 H 1 and A 2 H 2 – perpendiculars to the straight line Ox.
It is easy to see that right triangles OA 1 H 1 and OA 2 H 2 are equal in hypotenuse and two adjacent angles. From the equality of triangles and the location of points A 1 and A 2 on the unit circle, it becomes clear that if point A 1 has coordinates x and y, then point A 2 has coordinates −y and x. Then the definitions of sine and cosine allow us to write the equalities and 
, from which it follows that And . This proves the reduction formulas under consideration for any angle.
Considering that 
And 
(if necessary, see the article basic trigonometric identities), as well as the just proven formulas, we obtain and 
. So we proved the following two reduction formulas.
To prove reduction formulas with an argument, it is enough to represent it as , and then use the proven formulas and properties of trigonometric functions with opposite arguments. For example, .
All other reduction formulas are proved in a similar way on the basis of those already proven by double application. For example, it appears as 
, but as 
. And and - as and respectively.
Bibliography.
- Algebra: Textbook for 9th grade. avg. school/Yu. N. Makarychev, N. G. Mindyuk, K. I. Neshkov, S. B. Suvorova; Ed. S. A. Telyakovsky. - M.: Education, 1990. - 272 pp.: ill. - ISBN 5-09-002727-7
- Bashmakov M. I. Algebra and the beginnings of analysis: Textbook. for 10-11 grades. avg. school - 3rd ed. - M.: Education, 1993. - 351 p.: ill. - ISBN 5-09-004617-4.
- Algebra and the beginning of analysis: Proc. for 10-11 grades. general education institutions / A. N. Kolmogorov, A. M. Abramov, Yu. P. Dudnitsyn and others; Ed. A. N. Kolmogorov. - 14th ed. - M.: Education, 2004. - 384 pp.: ill. - ISBN 5-09-013651-3.
- Gusev V. A., Mordkovich A. G. Mathematics (a manual for those entering technical schools): Proc. allowance.- M.; Higher school, 1984.-351 p., ill.
Reduction formulas are relationships that allow you to go from sine, cosine, tangent and cotangent with angles `\frac (\pi)2 \pm \alpha`, `\pi \pm \alpha`, `\frac (3\pi) 2 \pm \alpha`, `2\pi \pm \alpha` to the same functions of the angle `\alpha`, which is located in the first quarter of the unit circle. Thus, the reduction formulas “lead” us to working with angles in the range from 0 to 90 degrees, which is very convenient.
All together there are 32 reduction formulas. They will undoubtedly come in handy during the Unified State Exam, exams, and tests. But let us immediately warn you that there is no need to memorize them! You need to spend a little time and understand the algorithm for their application, then it will not be difficult for you to derive the necessary equality at the right time.
First, let's write down all the reduction formulas:
For angle (`\frac (\pi)2 \pm \alpha`) or (`90^\circ \pm \alpha`):
`sin(\frac (\pi)2 - \alpha)=cos \ \alpha;` ` sin(\frac (\pi)2 + \alpha)=cos \ \alpha`
`cos(\frac (\pi)2 — \alpha)=sin \ \alpha;` ` cos(\frac (\pi)2 + \alpha)=-sin \ \alpha`
`tg(\frac (\pi)2 — \alpha)=ctg \ \alpha;` ` tg(\frac (\pi)2 + \alpha)=-ctg \ \alpha`
`ctg(\frac (\pi)2 — \alpha)=tg \ \alpha;` ` ctg(\frac (\pi)2 + \alpha)=-tg \ \alpha`
For angle (`\pi \pm \alpha`) or (`180^\circ \pm \alpha`):
`sin(\pi - \alpha)=sin \ \alpha;` ` sin(\pi + \alpha)=-sin \ \alpha`
`cos(\pi - \alpha)=-cos \ \alpha;` ` cos(\pi + \alpha)=-cos \ \alpha`
`tg(\pi - \alpha)=-tg \ \alpha;` ` tg(\pi + \alpha)=tg \ \alpha`
`ctg(\pi - \alpha)=-ctg \ \alpha;` ` ctg(\pi + \alpha)=ctg \ \alpha`
For angle (`\frac (3\pi)2 \pm \alpha`) or (`270^\circ \pm \alpha`):
`sin(\frac (3\pi)2 — \alpha)=-cos \ \alpha;` ` sin(\frac (3\pi)2 + \alpha)=-cos \ \alpha`
`cos(\frac (3\pi)2 — \alpha)=-sin \ \alpha;` ` cos(\frac (3\pi)2 + \alpha)=sin \ \alpha`
`tg(\frac (3\pi)2 — \alpha)=ctg \ \alpha;` ` tg(\frac (3\pi)2 + \alpha)=-ctg \ \alpha`
`ctg(\frac (3\pi)2 — \alpha)=tg \ \alpha;` ` ctg(\frac (3\pi)2 + \alpha)=-tg \ \alpha`
For angle (`2\pi \pm \alpha`) or (`360^\circ \pm \alpha`):
`sin(2\pi - \alpha)=-sin \ \alpha;` ` sin(2\pi + \alpha)=sin \ \alpha`
`cos(2\pi - \alpha)=cos \ \alpha;` ` cos(2\pi + \alpha)=cos \ \alpha`
`tg(2\pi - \alpha)=-tg \ \alpha;` ` tg(2\pi + \alpha)=tg \ \alpha`
`ctg(2\pi - \alpha)=-ctg \ \alpha;` ` ctg(2\pi + \alpha)=ctg \ \alpha`
You can often find reduction formulas in the form of a table where angles are written in radians:
To use it, we need to select the row with the function we need and the column with the desired argument. For example, to find out using a table what ` sin(\pi + \alpha)` will be equal to, it is enough to find the answer at the intersection of the row ` sin \beta` and the column ` \pi + \alpha`. We get ` sin(\pi + \alpha)=-sin \ \alpha`.
And the second, similar table, where angles are written in degrees:
Mnemonic rule for reduction formulas or how to remember them
As we already mentioned, there is no need to memorize all the above relationships. If you looked at them carefully, you probably noticed some patterns. They allow us to formulate a mnemonic rule (mnemonic - remember), with the help of which we can easily obtain any reduction formula.
Let us immediately note that to apply this rule you need to be good at identifying (or remembering) the signs of trigonometric functions in different quarters of the unit circle. 
The vaccine itself contains 3 stages:
- The function argument must be represented as `\frac (\pi)2 \pm \alpha`, `\pi \pm \alpha`, `\frac (3\pi)2 \pm \alpha`, `2\pi \ pm \alpha`, and `\alpha` is necessarily an acute angle (from 0 to 90 degrees).
- For the arguments `\frac (\pi)2 \pm \alpha`, `\frac (3\pi)2 \pm \alpha` the trigonometric function of the transformed expression changes to a cofunction, that is, the opposite (sine to cosine, tangent to cotangent and vice versa). For arguments `\pi \pm \alpha`, `2\pi \pm \alpha` the function does not change.
- The sign of the original function is determined. The resulting function on the right side will have the same sign.
To see how this rule can be applied in practice, let’s transform several expressions:
1. `cos(\pi + \alpha)`.
The function is not reversed. The angle `\pi + \alpha` is in the third quarter, the cosine in this quarter has a “-” sign, so the transformed function will also have a “-” sign.
Answer: ` cos(\pi + \alpha)= - cos \alpha`
2. `sin(\frac (3\pi)2 - \alpha)`.
According to the mnemonic rule, the function will be reversed. The angle `\frac (3\pi)2 - \alpha` is in the third quarter, the sine here has a “-” sign, so the result will also have a “-” sign.
Answer: `sin(\frac (3\pi)2 - \alpha)= - cos \alpha`
3. `cos(\frac (7\pi)2 - \alpha)`.
`cos(\frac (7\pi)2 - \alpha)=cos(\frac (6\pi)2+\frac (\pi)2-\alpha)=cos (3\pi+(\frac(\pi )2-\alpha))`. Let's represent `3\pi` as `2\pi+\pi`. `2\pi` is the period of the function.
Important: The functions `cos \alpha` and `sin \alpha` have a period of `2\pi` or `360^\circ`, their values will not change if the argument is increased or decreased by these values.
Based on this, our expression can be written as follows: `cos (\pi+(\frac(\pi)2-\alpha)`. Applying the mnemonic rule twice, we get: `cos (\pi+(\frac(\pi) 2-\alpha)= - cos (\frac(\pi)2-\alpha)= - sin \alpha`.
Answer: `cos(\frac (7\pi)2 - \alpha)=- sin \alpha`.
Horse rule
The second point of the mnemonic rule described above is also called the horse rule of reduction formulas. I wonder why horses?
So, we have functions with arguments `\frac (\pi)2 \pm \alpha`, `\pi \pm \alpha`, `\frac (3\pi)2 \pm \alpha`, `2\pi \ pm \alpha`, points `\frac (\pi)2`, `\pi`, `\frac (3\pi)2`, `2\pi` are key, they are located on the coordinate axes. `\pi` and `2\pi` are on the horizontal x-axis, and `\frac (\pi)2` and `\frac (3\pi)2` are on the vertical ordinate.
We ask ourselves the question: “Does a function change into a cofunction?” To answer this question, you need to move your head along the axis on which the key point is located.
That is, for arguments with key points located on the horizontal axis, we answer “no” by shaking our heads to the sides. And for corners with key points located on the vertical axis, we answer “yes” by nodding our heads from top to bottom, like a horse :)
We recommend watching a video tutorial in which the author explains in detail how to remember reduction formulas without memorizing them.
Practical examples of using reduction formulas
The use of reduction formulas begins in grades 9 and 10. Many problems using them were submitted to the Unified State Exam. Here are some of the problems where you will have to apply these formulas:
- problems to solve a right triangle;
- transformation of numeric and alphabetic trigonometric expressions, calculation of their values;
- stereometric tasks.
Example 1. Calculate using reduction formulas a) `sin 600^\circ`, b) `tg 480^\circ`, c) `cos 330^\circ`, d) `sin 240^\circ`.
Solution: a) `sin 600^\circ=sin (2 \cdot 270^\circ+60^\circ)=-cos 60^\circ=-\frac 1 2`;
b) `tg 480^\circ=tg (2 \cdot 270^\circ-60^\circ)=ctg 60^\circ=\frac(\sqrt 3)3`;
c) `cos 330^\circ=cos (360^\circ-30^\circ)=cos 30^\circ=\frac(\sqrt 3)2`;
d) `sin 240^\circ=sin (270^\circ-30^\circ)=-cos 30^\circ=-\frac(\sqrt 3)2`.
Example 2. Having expressed cosine through sine using reduction formulas, compare the numbers: 1) `sin \frac (9\pi)8` and `cos \frac (9\pi)8`; 2) `sin \frac (\pi)8` and `cos \frac (3\pi)10`.
Solution: 1)`sin \frac (9\pi)8=sin (\pi+\frac (\pi)8)=-sin \frac (\pi)8`
`cos \frac (9\pi)8=cos (\pi+\frac (\pi)8)=-cos \frac (\pi)8=-sin \frac (3\pi)8`
`-sin \frac (\pi)8> -sin \frac (3\pi)8`
`sin \frac (9\pi)8>cos \frac (9\pi)8`.
2) `cos \frac (3\pi)10=cos (\frac (\pi)2-\frac (\pi)5)=sin \frac (\pi)5`
`sin \frac (\pi)8 `sin \frac (\pi)8 Let us first prove two formulas for the sine and cosine of the argument `\frac (\pi)2 + \alpha`: ` sin(\frac (\pi)2 + \alpha)=cos \ \alpha` and ` cos(\frac (\ pi)2 + \alpha)=-sin \ \alpha`. The rest are derived from them. Let's take a unit circle and point A on it with coordinates (1,0). Let after turning to Coming from the definition of tangent and cotangent, we obtain ` tan(\frac (\pi)2 + \alpha)=\frac (sin(\frac (\pi)2 + \alpha))(cos(\frac (\pi)2 + \alpha))=\frac (cos \alpha)(-sin \alpha)=-ctg \alpha` and ` сtg(\frac (\pi)2 + \alpha)=\frac (cos(\frac (\ pi)2 + \alpha))(sin(\frac (\pi)2 + \alpha))=\frac (-sin \alpha)(cos \alpha)=-tg \alpha`, which proves the reduction formulas for tangent and the cotangent of the angle `\frac (\pi)2 + \alpha`. To prove formulas with the argument `\frac (\pi)2 - \alpha`, it is enough to represent it as `\frac (\pi)2 + (-\alpha)` and follow the same path as above. For example, `cos(\frac (\pi)2 - \alpha)=cos(\frac (\pi)2 + (-\alpha))=-sin(-\alpha)=sin(\alpha)`. The angles `\pi + \alpha` and `\pi - \alpha` can be represented as `\frac (\pi)2 +(\frac (\pi)2+\alpha)` and `\frac (\pi) 2 +(\frac (\pi)2-\alpha)` respectively. And `\frac (3\pi)2 + \alpha` and `\frac (3\pi)2 - \alpha` as `\pi +(\frac (\pi)2+\alpha)` and `\pi +(\frac (\pi)2-\alpha)`. They belong to the trigonometry section of mathematics. Their essence is to reduce trigonometric functions of angles to a “simple” form. Much can be written about the importance of knowing them. There are already 32 of these formulas! Don’t be alarmed, you don’t need to learn them, like many other formulas in a math course. There is no need to fill your head with unnecessary information, you need to remember the “keys” or laws, and remembering or deriving the required formula will not be a problem. By the way, when I write in articles “... you need to learn!!!” - this means that it really needs to be learned. If you are not familiar with reduction formulas, then the simplicity of their derivation will pleasantly surprise you - there is a “law” with the help of which this can be easily done. And you can write any of the 32 formulas in 5 seconds. I will list only some of the problems that will appear on the Unified State Exam in mathematics, where without knowledge of these formulas there is a high probability of failing in solving them. For example: – problems for solving a right triangle, where we are talking about the external angle, and problems for internal angles, some of these formulas are also necessary. – tasks on calculating the values of trigonometric expressions; converting numerical trigonometric expressions; converting literal trigonometric expressions. – problems on the tangent and the geometric meaning of the tangent, a reduction formula for the tangent is required, as well as other problems. – stereometric problems, in the course of solving it is often necessary to determine the sine or cosine of an angle that lies in the range from 90 to 180 degrees. And these are just those points that relate to the Unified State Exam. And in the algebra course itself there are many problems, the solution of which simply cannot be done without knowledge of reduction formulas. So what does this lead to and how do the specified formulas make it easier for us to solve problems? For example, you need to determine the sine, cosine, tangent, or cotangent of any angle from 0 to 450 degrees: the alpha angle ranges from 0 to 90 degrees * * *
So, it is necessary to understand the “law” that works here: 1. Determine the sign of the function in the corresponding quadrant. Let me remind you: 2. Remember the following: function changes to cofunction
function does not change to cofunction
What does the concept mean - a function changes to a cofunction?
Answer: sine changes to cosine or vice versa, tangent to cotangent or vice versa.
That's all! Now, according to the presented law, we will write down several reduction formulas ourselves: This angle lies in the third quarter, the cosine in the third quarter is negative. We don’t change the function to a cofunction, since we have 180 degrees, which means: The angle lies in the first quarter, the sine in the first quarter is positive. We do not change the function to a cofunction, since we have 360 degrees, which means: Here is another additional confirmation that the sines of adjacent angles are equal: The angle lies in the second quarter, the sine in the second quarter is positive. We do not change the function to a cofunction, since we have 180 degrees, which means: Work through each formula mentally or in writing, and you will be convinced that there is nothing complicated. ***
In the article on the solution, the following fact was noted - the sine of one acute angle in a right triangle is equal to the cosine of another acute angle in it. How not to memorize reduction formulas. When solving trigonometric equations or performing trigonometric transformations, the first step is to minimize the number of different arguments of trigonometric functions. To do this, you need to bring all the angles to the angles of the first quarter, using reduction formulas. I want to introduce you to a mnemonic rule that allows you to avoid memorizing. This rule is jokingly called the "Horse Rule". In this VIDEO TUTORIAL I will tell you how to use this rule: reduce the trigonometric function of an arbitrary angle to the angle of the first quarter, freeing yourself from the need to remember reduction formulas: So, " horse rule
" sounds like this: If we plot the angle from vertical axis, the horse says “yes” (we nod our head along the OY axis) and the reducible function changes its name: sine to cosine, cosine to sine, tangent to cotangent, cotangent to tangent.
If we plot the angle from horizontal axis, the horse says “no” (we nod our head along the OX axis) and the reduced function does not change its name.
The sign on the right side of the equality coincides with the sign of the reducible function on the left side of the equality.
Here are some examples of using reduction formulas: 1
. Find the meaning of the expression: 1. Select the whole part in the fraction: 2. Since the period of the function is equal to , let’s highlight the “idle speed”: Now our argument is in the range from zero to , and it's time to apply the "horse rule": To get to the point corresponding to the rotation angle by , we first make a rotation by radians, and then from this point we plot an angle of radians: 2
. Find the meaning of the expression: Let's look at each function separately: We first rotate by a radian and then make an angle of 1 radian from the vertical axis in the negative direction and end up in the third quarter: There are two rules for using reduction formulas. 1. If the angle can be represented as (π/2 ±a) or (3*π/2 ±a), then function name changes sin to cos, cos to sin, tg to ctg, ctg to tg. If the angle can be represented in the form (π ±a) or (2*π ±a), then The function name remains unchanged. Look at the picture below, it shows schematically when you should change the sign and when not. 2. The rule “as you were, so you remain.” The sign of the reduced function remains the same. If the original function had a plus sign, then the reduced function also has a plus sign. If the original function had a minus sign, then the reduced function also has a minus sign. The figure below shows the signs of the basic trigonometric functions depending on the quarter. Calculate Sin(150˚) Let's use the reduction formulas: Sin(150˚) is in the second quarter; from the figure we see that the sign of sin in this quarter is equal to +. This means that the given function will also have a plus sign. We applied the second rule. Now 150˚ = 90˚ +60˚. 90˚ is π/2. That is, we are dealing with the case π/2+60, therefore, according to the first rule, we change the function from sin to cos. As a result, we get Sin(150˚) = cos(60˚) = ½. If desired, all reduction formulas can be summarized in one table. But it’s still easier to remember these two rules and use them.
angle `\alpha` it will go to point `A_1(x, y)`, and after turning by angle `\frac (\pi)2 + \alpha` to point `A_2(-y, x)`. Dropping the perpendiculars from these points to the line OX, we see that the triangles `OA_1H_1` and `OA_2H_2` are equal, since their hypotenuses and adjacent angles are equal. Then, based on the definitions of sine and cosine, we can write `sin \alpha=y`, `cos \alpha=x`, `sin(\frac (\pi)2 + \alpha)=x`, `cos(\frac (\ pi)2 + \alpha)=-y`. Where can we write that ` sin(\frac (\pi)2 + \alpha)=cos \alpha` and ` cos(\frac (\pi)2 + \alpha)=-sin \alpha`, which proves the reduction formulas for sine and cosine angles `\frac (\pi)2 + \alpha`.
We plotted the angle from the horizontal axis (the horse says “no”) - it does not change its name, the angle is located in the third quarter, in which the cosine is negative, therefore the reduced function is negative. We get:
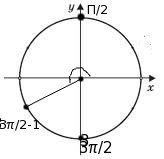Consequently, the reducible function changes its name, the reducible function is greater than zero (the tangent of the third quarter angle is greater than zero): 
.
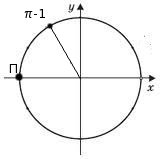First we make a turn by a radian, and then from this point we move by 1 radian in a negative direction. We set aside an angle of 1 radian from the horizontal axis (the sine does not change its name) and find ourselves in the second quarter, in which the sine is greater than zero:
Need help with your studies?
Previous topic:
